
10.Moment of inertia or mass moment of inertia is the resistance of a rigid body to change in its angular velocity or we can say, resistance to angular acceleration, when a net external torque acts on it (similar to resistance offered by mass of a particle to acceleration, when a net force acts on it). If is a point in the plane of an area and distant from the centroid of the area as shown in Fig. The parallel axis theorem also hold for the polar moment of inertia. When opposed to a solid shaft, a hollow shaft transmits greater power (both of same mass). Moment of Inertia Example 3: Hollow shaft. The flywheel’s Moment Of Inertia is extremely large, which aids in energy storage. A flywheel is a large mass situated on an engine’s crankshaft. As the size of these particles tends to zero, it can be thought of as made up of small cubes with dimensions w. Moment of Inertia Example 2: FLYWHEEL of an automobile.
which is the sum of all the elemental particles masses multiplied by their distance from the rotational axis squared. The Moment of inertia for hollow rectangular section formula is defined as a geometrical property of an area which reflects how its points are distributed with regard to an arbitrary axis and is represented as I circular ((B outer (L rectangle 3))-(B inner (L inner 3)))/12 or M. Where is the distance between the two parallel axes. The moment of inertia of the shape is given by the equation. If is an axis crossing, and a parallel axis to as shown in Fig. Įquation 10.7 can be written for any two parallel axes with one crossing the centroid of the area.
#Moment of inertia formula plus#
Which reads the moment of inertia about an axis is equal to the moment of inertia about a parallel axis that crosses the centroid of, plus the product of area and the square distance between and. Planar and polar moments of inertia both fall under the.
Planar and polar moments of inertia formulas. torque (moment of inertia) (angular acceleration) I torque, around a defined axis (Nm) I moment of inertia (kgm 2) angular acceleration (radians/s 2) Torque Formula Questions: 1) The moment of inertia of a solid disc is, where M is the mass of the disc, and R is the radius. The term equals zero because and (measured from the axis) because passes through the centroid. Mass moment of inertia is important for motor sizing, where the inertia ratio the ratio of the load inertia to the motor inertia plays a significant role in determining how well the motor can control the load’s acceleration and deceleration. If is a differential element of the area, its (perpendicular) distance to the axis can be written as where is the distance between the two parallel axes shown in Fig. 10.9 Terms involved in deriving the parallel axis theorem.
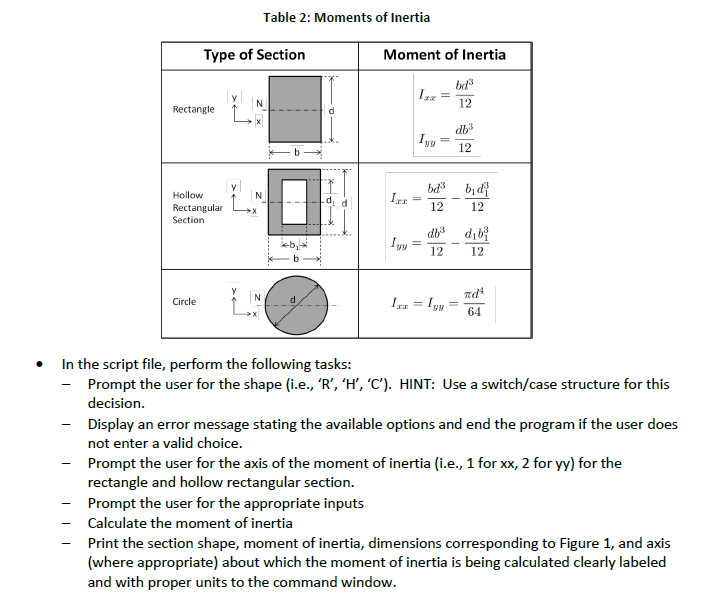
We have a comprehensive article explaining the approach to solving the moment of inertia. Equilibrium of Particles and Rigid Bodies The moment of inertia of is given by: Where we have: m: mass. The moment of inertia can be derived as getting the moment of inertia of the parts and applying the transfer formula: I I 0 + Ad 2.Simplification of force and couple systems.Vector operations using Cartesian vector notation.Vector operations using the parallelogram rule and trigonometry.


 0 kommentar(er)
0 kommentar(er)
